Remember that overwhelming feeling when you first encountered equations with parentheses? It felt like a whole new level of math! That’s where the distributive property came in, and thankfully, it simplified everything. It’s like a magical tool that allows us to break down complex equations, piece by piece, and solve them with confidence.
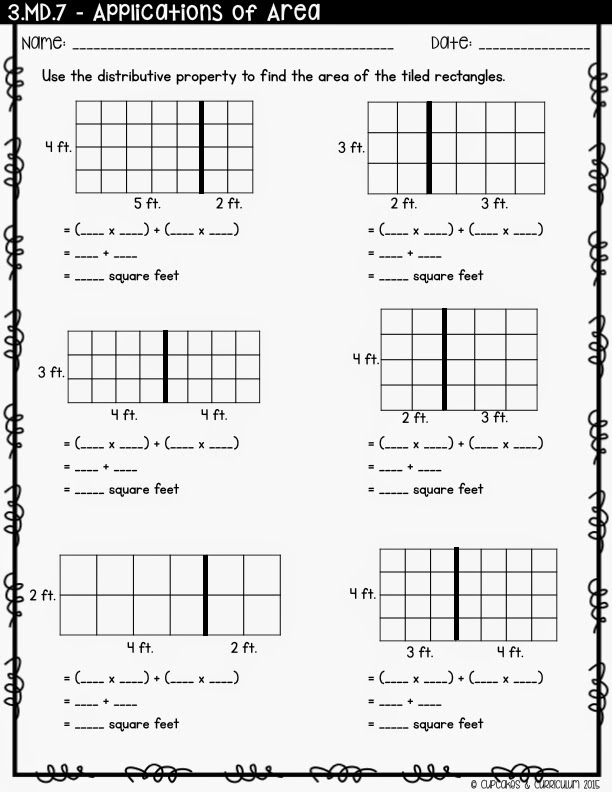
Image: www.cupcakesncurriculum.com
In this lesson, we’re diving into the distributive property, uncovering its secrets, and exploring why it’s a fundamental concept in mathematics. We’ll break down how it works, why it’s so important, and show you how it can make your homework a breeze.
Unveiling the Magic of the Distributive Property
The distributive property is a powerful tool in mathematics. It allows us to multiply a sum by a number by multiplying each addend separately and then adding the products.
Imagine you have a group of 3 friends, and each friend wants to buy 2 apples and 1 orange. You can figure out the total number of fruits they want by multiplying the number of friends (3) by the number of apples (2) and the number of oranges (1) separately, and then adding the products. That’s exactly what the distributive property does!
Understanding the Distributive Property
The distributive property can be represented using the following formula:
a(b + c) = ab + ac
Where:
- a represents the number being multiplied by the sum
- b and c represent the numbers inside the parentheses
Here’s a breakdown of how the distributive property works:
- Multiply the number outside the parentheses by each term inside the parentheses. In our formula, this means multiplying a by both b and c.
- Add the resulting products. This gives you the final result of the multiplication.

Image: davida.davivienda.com
Examples of the Distributive Property
Let’s look at a few examples to solidify our understanding:
- 3(x + 2)
Apply the distributive property: 3 * x + 3 * 2
Simplify: 3x + 6 - -2(4y – 5)
Apply the distributive property: -2 * 4y + -2 * -5
Simplify: -8y + 10 - 5(2a + 3b – 1)
Apply the distributive property: 5 * 2a + 5 * 3b + 5 * -1
Simplify: 10a + 15b – 5
Why the Distributive Property Matters
The distributive property is a foundational concept in algebra and beyond. Why? Let’s explore:
- Simplifying expressions: The distributive property allows us to break down complex expressions into simpler forms, making them easier to work with. This is especially helpful when solving for variables or manipulating equations.
- Solving equations: The distributive property is a crucial tool in solving equations involving parentheses. It allows us to isolate variables and find their values effectively.
- Building a foundation for higher math: Understanding the distributive property is essential for tackling more advanced math topics like factoring, quadratic equations, and calculus.
Tips and Expert Advice
Here are some tips to enhance your understanding of the distributive property and make it easy to apply:
- Visualize the process: When applying the distributive property, imagine “distributing” the number outside the parentheses to each term inside. This visual representation can help you remember the steps.
- Practice with various examples: The more you practice using the distributive property with different equations, the more confident you’ll become. Work through numerous examples to solidify your understanding of the concept. Look for examples in your textbook, online resources, or even create your own!
- Break down complex problems: When you encounter expressions with multiple parentheses, break them down step by step. Start with one set of parentheses, apply the distributive property, and then continue with the next set. This approach prevents confusion and makes it easier to manage the calculations.
Remember, like any skill, mastering the distributive property takes practice. Stay persistent, and you’ll find yourself confidently applying it to a wide range of problems.
Frequently Asked Questions (FAQs)
Let’s address some common questions about the distributive property:
Q: Can the distributive property be used with subtraction inside the parentheses?
A: Absolutely! The distributive property holds true for both addition and subtraction. For example, 2(x – 3) would be distributed as 2 * x – 2 * 3.
Q: Is there a specific order in which I should apply the distributive property when multiple sets of parentheses are involved?
A: While the order technically doesn’t matter, it’s often easier to apply the distributive property from left to right, working your way through the parentheses one at a time.
Q: What if there are variables outside the parentheses as well?
A: The distributive property still applies, and you simply multiply the variables together as well. For example, x(2y + 3) would be distributed as x * 2y + x * 3 which simplifies to 2xy + 3x.
My Homework Lesson 7 The Distributive Property
Conclusion
The distributive property is a fundamental concept in mathematics that helps us simplify expressions, solve equations, and tackle complex problems. By understanding its mechanics and practicing its application, you can confidently use it in your homework and beyond. Remember to visualize the process, practice with various examples, and break down complex problems into smaller steps.
Are you ready to conquer your homework using the power of the distributive property? What other math topics are you interested in exploring? Share your thoughts below!






