Have you ever felt a pang of anxiety when you see a long, complicated equation in your Financial Algebra textbook? You’re not alone! Many students struggle with the concepts covered in Chapter 2, which delves into the realm of solving equations and inequalities. While these mathematical tools might initially seem intimidating, they unlock a deeper understanding of financial concepts and lay the groundwork for tackling more complex problems later on.
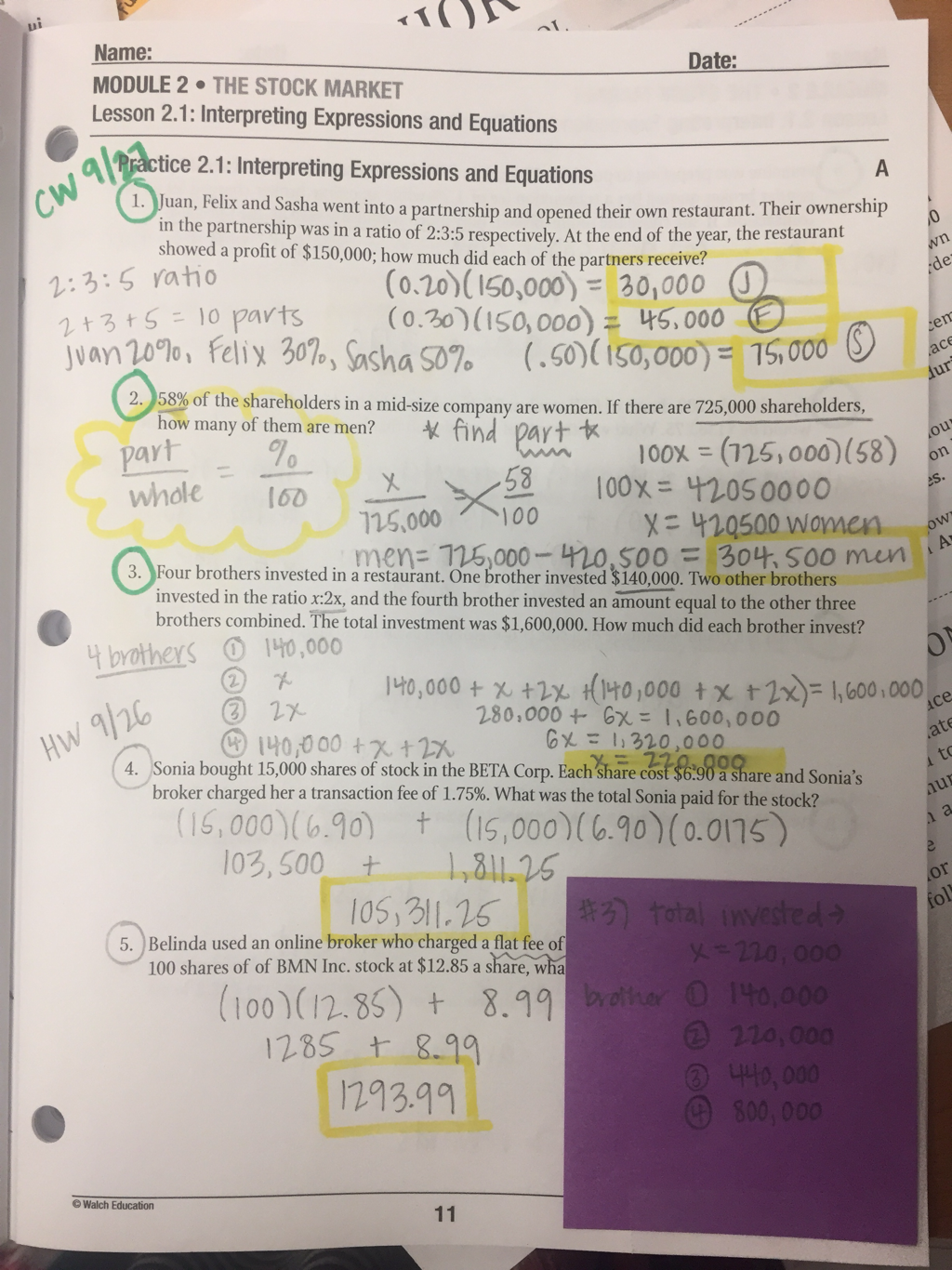
Image: ahscalculus.weebly.com
But fear not! This comprehensive guide will walk you through the key concepts of Chapter 2, equip you with the tools to solve equations and inequalities, and provide valuable insights into why these skills are critical for your financial literacy journey. We’ll also explore how these fundamental concepts are applied in real-world scenarios, making them less abstract and more relevant to your daily life.
Understanding the Basics: Equations and Inequalities
Equations: Finding the Balance
An equation is like a carefully balanced scale. It expresses the equality of two mathematical expressions. Imagine a scale where one side represents the left-hand side of the equation, and the other side represents the right-hand side. To solve an equation, you’re essentially trying to find the value of the unknown variable that makes the scale perfectly balanced.
For instance, consider the equation 2x + 5 = 11. Here, “x” is the unknown variable. To solve for “x,” you need to manipulate the equation to isolate “x” on one side. Here’s how:
-
Subtract 5 from both sides: This ensures that the scale remains balanced.
2x + 5 – 5 = 11 – 5
2x = 6 -
Divide both sides by 2: This isolates “x” on one side.
2x / 2 = 6 / 2
x = 3
Therefore, the solution to the equation 2x + 5 = 11 is x = 3. This means that if you substitute “3” for “x” in the original equation, both sides will be equal.
Inequalities: Showing the Differences
Unlike equations that represent equality, inequalities show a difference in value. They use symbols like < (less than), > (greater than), ≤ (less than or equal to), and ≥ (greater than or equal to). Solving inequalities involves finding the range of values for the unknown variable that satisfy the inequality.
Take the example of 3x – 2 < 7. To solve this inequality, we follow similar steps as with equations, but we need to be mindful of the direction of the inequality sign.
-
Add 2 to both sides:
3x – 2 + 2 < 7 + 2
3x < 9 -
Divide both sides by 3: Remember to keep the inequality sign pointing in the same direction.
3x / 3 < 9 / 3
x < 3
Therefore, the solution to the inequality 3x – 2 < 7 is x < 3. This means any value of “x” less than 3 will satisfy the inequality.
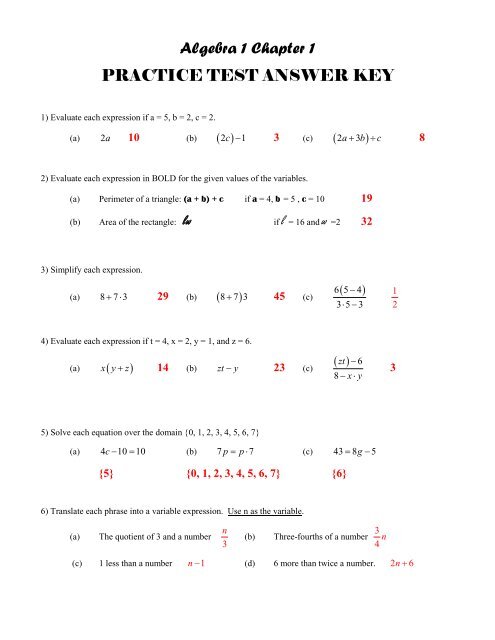
Image: juriemmiee.blogspot.com
Navigating Financial Algebra Chapter 2: Key Concepts
1. Solving Linear Equations
Chapter 2 focuses on solving linear equations, which are equations where the variables have an exponent of 1. These equations often appear in financial scenarios like calculating interest earned on savings accounts or figuring out how much to invest to reach a specific goal.
Here are some common strategies for solving linear equations:
- Combining like terms: Simplify the equation by combining terms with the same variable or constant terms. For example, in the equation 2x + 3y – 4x + 5y = 10, we can combine the “x” terms and the “y” terms to get -2x + 8y = 10.
- Using the distributive property: When a term multiplies a sum or difference within parentheses, distribute the term to each element inside the parentheses. For example, 2(x + 3) = 2x + 6.
- Isolating the variable: Perform operations on both sides of the equation to isolate the variable on one side. Remember to keep the equation balanced by applying the same operation to both sides.
2. Solving Linear Inequalities
Similar to linear equations, Chapter 2 delves into solving linear inequalities. These inequalities are used to represent financial constraints or comparisons, such as finding the maximum amount of money you can spend while staying within a budget.
Solving linear inequalities involves using similar techniques as solving linear equations, but with a key difference: **the direction of the inequality sign**. When multiplying or dividing both sides of an inequality by a negative number, you must reverse the direction of the inequality sign.
3. Graphing Linear Equations and Inequalities
Chapter 2 also introduces the concept of graphing linear equations and inequalities. This visual representation allows you to understand the relationship between variables and identify solutions to equations and inequalities.
To graph a linear equation, we need two points. One way to find these points is to use the *x* and *y* intercepts. The *x*-intercept is the point where the graph crosses the *x*-axis, and the *y*-intercept is the point where the graph crosses the *y*-axis. You can also find points on the graph by substituting different values for *x* and solving for *y* (or vice versa).
To graph a linear inequality, we start by graphing the corresponding linear equation. Then, we shade the region that satisfies the inequality. If the inequality is *strictly less than* or *strictly greater than*, we use a dashed line to indicate that the points on the line are *not* included in the solution. If the inequality is *less than or equal to* or *greater than or equal to*, we use a solid line to indicate that the points on the line *are* included in the solution.
Practical Applications: Bringing Equations to Life
The concepts you learn in Chapter 2 of Financial Algebra are far from theoretical exercises. They have real-world applications that can empower you to make sound financial decisions.
1. Budgeting and Financial Planning
Equations and inequalities are essential tools for creating and managing a budget. You can use linear equations to calculate expenses, savings goals, and income projections. Inequalities come into play when you need to set limits on spending or determine how much income is needed to meet specific financial objectives.
For example, suppose you want to save $500 per month. You can set up the following equation:
Total Savings = Monthly Savings * Number of Months
To achieve your goal of $500 per month, you can plug in different values for the “Number of Months” and calculate the corresponding “Total Savings.” This equation can help you track your progress toward your savings target.
2. Interest Calculations and Loans
Understanding linear equations is crucial for calculating interest earned on savings accounts or interest accrued on loans. These equations help you determine how much money you’ll earn or owe over time, based on the interest rate and the principal amount.
For instance, the simple interest formula is:
Interest = Principal Rate Time
This equation helps you compute the interest earned or owed for a given principal amount, rate, and time period. By understanding this equation, you can compare different interest rates and choose the most favorable financial products.
3. Investment Decisions and Portfolio Management
Linear equations and inequalities are employed in investment decision-making. They allow you to assess different investment options and determine the potential returns on your investments. Furthermore, inequalities are helpful for managing investment portfolios and staying within risk tolerance limits.
Take the example of investing in stocks. You can use equations to calculate the potential return on investment based on expected growth rates and dividends. Inequalities can be used to ensure that the portfolio remains balanced and meets your desired risk level.
Mastering Chapter 2: Tips for Success
Here are some strategies for mastering the concepts covered in Financial Algebra Chapter 2:
- Practice, practice, practice: The best way to understand and retain these concepts is through consistent practice. Work through numerous problems, both from the textbook and online resources, to solidify your understanding.
- Visualize the equations: Use graphs and diagrams to visualize the relationships between variables. This will make the equations more intuitive and easier to comprehend.
- Apply the concepts to real-world scenarios: Connect the mathematical concepts to your own financial goals and situations. This will strengthen your understanding and motivation.
- Seek help when needed: Don’t hesitate to reach out to your teacher or a tutor if you’re struggling with a particular concept.
- Utilize online resources: Explore online tutorials, video explanations, and interactive exercises to supplement your textbook materials.
Financial Algebra Chapter 2 Test Answers
Conclusion: Unleashing Your Financial Potential
Mastering the concepts in Financial Algebra Chapter 2 is a key step in your journey to financial literacy. By understanding how to solve equations and inequalities, you’ll be equipped with powerful tools to analyze financial situations, make informed decisions, and achieve your financial goals. So, embrace the challenge, practice diligently, and watch your financial knowledge and confidence flourish.






