Have you ever wondered how much energy a speeding train possesses or how much work is done when lifting a heavy object? These are just a few examples of the real-world applications of work, power, and energy concepts, which are fundamental principles in physics. Understanding these concepts is crucial for anyone who wants to delve deeper into the workings of the physical world around us. But let’s be honest, grasping these concepts can be a bit challenging, especially when tackling complex problems. That’s where our comprehensive guide, “Work, Power, and Energy Problems with Solutions PDF,” comes in!

Image: www.scribd.com
This guide is designed to be your complete go-to resource for mastering work, power, and energy problems. We will guide you through the essential definitions, formulas, and problem-solving strategies, making the otherwise daunting task of solving physics problems a breeze. By the end of this guide, you’ll gain a deeper understanding of these concepts and develop the confidence to tackle any related question.
Understanding the Fundamentals of Work, Power, and Energy
Work: The Effort of Motion
In physics, work is a measure of the force applied to an object over a certain distance. It is defined as the product of force and displacement, W = F * d, where W represents work, F represents force, and d represents displacement. The key point here is that the force must cause a displacement to be considered work. For example, if you push against a wall, you are applying force, but no work is being done as the wall doesn’t move.
Power: The Rate of Work
Power is the rate at which work is done or the rate at which energy is transferred. It is calculated by dividing the work done by the time taken, P = W / t, where P represents power, W represents work, and t represents time. Imagine you have to move a box upstairs. Whether you carry it slowly or quickly, the work done is the same. However, the power exerted is different because it depends on how fast you move the box.
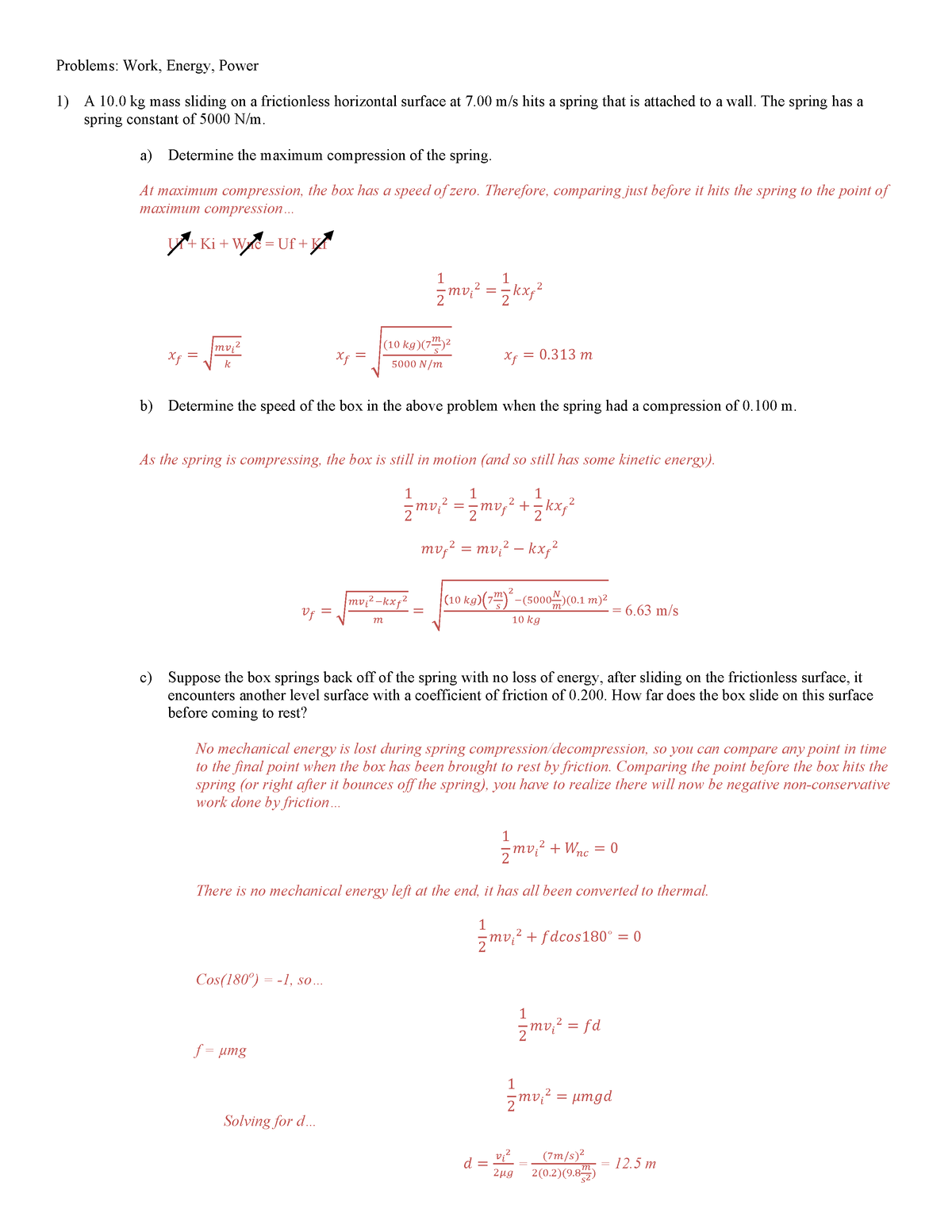
Image: www.studocu.com
Energy: The Capacity to do Work
Energy is the ability to do work. Like work, energy is expressed in joules (J). There are different forms of energy, including:
- Kinetic energy: The energy associated with the motion of an object, calculated as KE = 1/2 * mv², where KE represents kinetic energy, m represents mass, and v represents velocity.
- Potential energy: The energy an object possesses due to its position or configuration; there are different types, such as gravitational potential energy, which is dependent on height, and elastic potential energy, which is related to the stretching or compression of a spring.
Real-World Applications: Where Work, Power, and Energy Come Alive
The concepts of work, power, and energy are not just confined to textbook problems. They play a crucial role in numerous aspects of our daily lives. For instance, understanding these principles is essential in fields like engineering, where calculations are needed to design machines and structures, and in sports, where athletes strive to maximize their power output for optimal performance.
Engineering Marvels: From Bridges to Power Plants
Engineering feats, such as the construction of bridges, skyscrapers, and power plants, rely heavily on the application of work, power, and energy principles. Calculating the amount of work required to lift heavy building materials, determining the power output of engines, and assessing the energy efficiency of power plants are all tasks that engineers must master.
Sports Science: Optimizing Performance
In the realm of sports, understanding work, power, and energy can help athletes optimize their performance. Coaches use these concepts to analyze athletes’ movements, tailor training programs, and measure progress. For example, they can calculate the power output of a sprinter during a race or the energy expenditure of a marathon runner.
Practice Makes Perfect: Dive Into Problem-Solving Strategies
To truly grasp these concepts, it’s crucial to delve into solving problems using real-world examples and practical scenarios. This will help you apply the theoretical knowledge and develop a deeper understanding of their practical applications.
The Power of Problem-Solving: A Step-by-Step Approach
Here’s a breakdown of a step-by-step approach to solving work, power, and energy problems:
- Identify the known quantities: Carefully read the problem statement and identify the given values, such as mass, velocity, distance, time, or force.
- Determine the unknown quantity: Decide what you need to find, whether it’s work, power, potential energy, kinetic energy, or any other related quantity.
- Select the appropriate formula: Choose the relevant formula based on the problem’s context and the quantities involved.
- Substitute the values and solve: Plug in the known values into the selected formula and perform the necessary calculations to find the unknown quantity.
- Check the units and answer: Ensure the units of your answer are consistent with the units of the given quantities. Make sense of the answer in relation to the problem’s context.
Mastering the Art of Problem-Solving: Examples and Solutions
Let’s practice applying these problem-solving strategies with a few examples:
Example 1: Work Done by a Force
A person pushes a box across a smooth floor with a constant force of 50 N. If the box moves 3 meters, what is the work done by the person?
Solution:
- Known quantities: Force (F) = 50 N, Displacement (d) = 3 m
- Unknown quantity: Work (W)
- Formula: W = F * d
- Substitute and solve: W = 50 N * 3 m = 150 J
- Units and answer: The work done is 150 joules.
Example 2: Power of a Motor
A motor lifts a 100 kg object to a height of 5 meters in 2 seconds. What is the power output of the motor?
Solution:
- Known quantities: Mass (m) = 100 kg, Height (h) = 5 m, Time (t) = 2 s
- Unknown quantity: Power (P)
- Formulas:
- Work (W) = Potential Energy (PE) = mgh (where g is the acceleration due to gravity, approximately 9.8 m/s²)
- Power (P) = W / t
- Substitute and solve:
- W = mgh = 100 kg * 9.8 m/s² * 5 m = 4900 J
- P = W / t = 4900 J / 2 s = 2450 W
- Units and answer: The power output of the motor is 2450 watts.
Work Power And Energy Problems With Solutions Pdf
https://youtube.com/watch?v=BzNIVsE–QE
Unlocking Success: The Value of Our PDF Guide
Our “Work, Power, and Energy Problems with Solutions PDF” offers a wealth of knowledge and practical examples to guide you through the intricacies of these fundamental physics concepts. It is a valuable resource for students, teachers, and anyone seeking a deeper understanding of work, power, and energy.
By downloading our PDF guide, you gain access to a comprehensive collection of problems with detailed step-by-step solutions, covering various scenarios and applications. This resource is designed to enhance your problem-solving skills, build confidence in your understanding, and equip you with the tools needed to excel in your academic endeavors or in your pursuit of real-world applications.
Don’t hesitate to download our “Work, Power, and Energy Problems with Solutions PDF” today and embark on your journey towards mastery! Remember, practice is key, so make use of the examples provided and try solving additional problems on your own. The more you practice, the more confident and skilled you will become in conquering the world of work, power, and energy.






