Ever wondered how airplanes stay aloft, how bridges stand strong, or how architects design stunning buildings? The answer, in part, lies in the fascinating world of angles! And understanding angles isn’t just for engineers and architects, it’s a fundamental concept that permeates our everyday lives, from the way we hold our phones to the angles at which we kick a soccer ball. This guide will delve into the fascinating world of angles, specifically focusing on the key concepts that students in grades 1-4 need to master, along with some effective intervention strategies for those who need a little extra help.
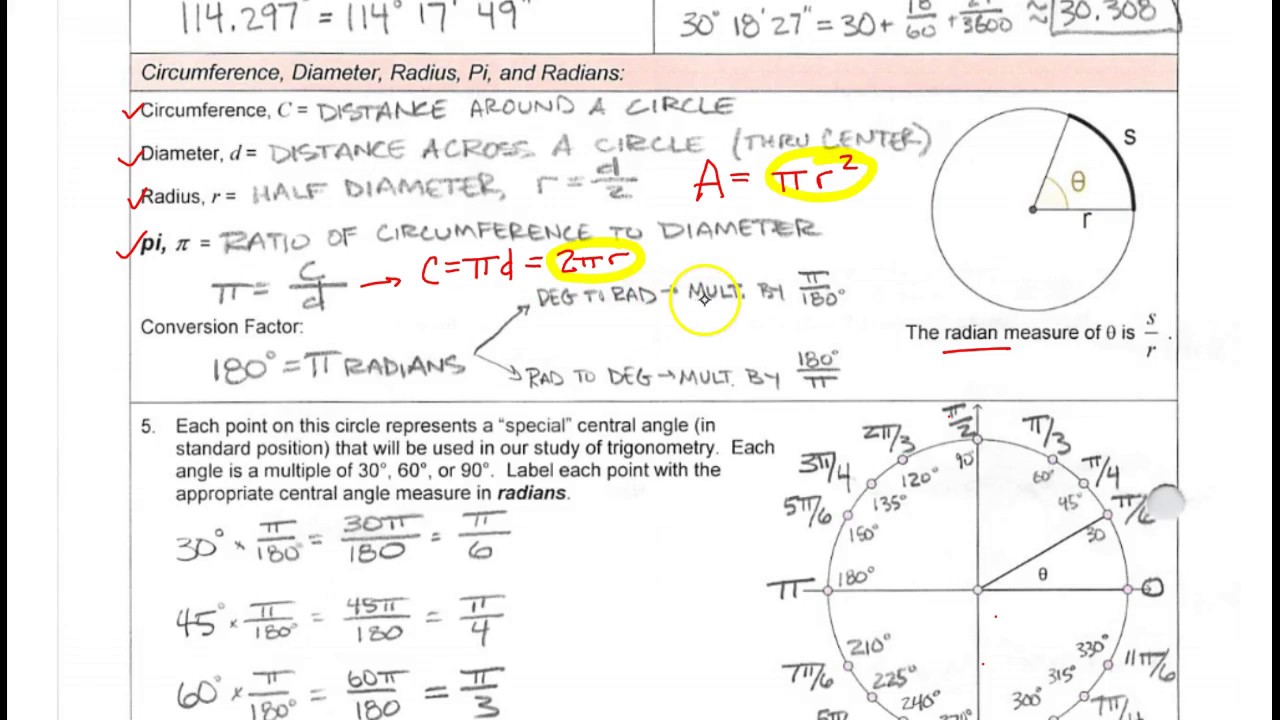
Image: www.youtube.com
Angles are a fundamental part of geometry. They are formed by two rays that share a common endpoint. This endpoint is called the vertex of the angle. Angles can be measured and classified based on their size, and understanding this is crucial for developing spatial reasoning skills.
The Building Blocks of Angles: Definitions and Concepts
What exactly is an angle?
Imagine you’re holding a piece of paper. Now, fold it in half. The crease you made is like an angle. It represents the space between two straight lines where they meet. That meeting point is called the vertex. We can measure an angle by looking at how much that space opens up.
Classifying Angles: Friends who come in different sizes
Just like people, angles come in different sizes. To help you understand those sizes, we group them into different categories:
- **Right angles:** These are the most well-known angles, forming a perfect corner like the corner of a square or the hand of a clock at 3 o’clock. They measure exactly 90 degrees.
- **Acute angles:** These angles are smaller than right angles. Think of them as a tiny slice of pizza; they are “cute” and less than 90 degrees.
- **Obtuse angles:** These angles are bigger than right angles but smaller than a straight line. Think of a yawning mouth; they are “obtusely” open, measuring more than 90 degrees but less than 180 degrees.
- **Straight angles:** These angles are perfectly flat, like a ruler or a straight line. They measure 180 degrees.
Image: www.youtube.com
Making Angles Concrete: Real-World Applications
Angles are more than just abstract mathematical concepts; they have a huge impact on our world.
Imagine the world without angles:
- **Architecture:** No more stunning skyscrapers or beautiful homes – just wobbly structures that are unsafe. Architects use angles to create stability and aesthetic appeal. Think of the slanted roof of a house or the pointed arches of a cathedral.
- **Transportation:** Forget about driving your car or flying in a plane! Angles are crucial for car design, making sure the car can turn corners safely. In aircraft, angles are essential for creating lift, allowing planes to soar through the sky.
- **Sports:** From the trajectory of a basketball shot to the perfect swing of a golf club, angles are everywhere in sports. Athletes use angles to achieve maximum power and accuracy.
- **Art:** Look at any painting, sculpture, or photograph. You’ll see angles used to create perspective and depth, guiding the viewer’s eye through the artwork.
Helping Students Thrive: Intervention Strategies for Angle Measures
Sometimes, students might struggle with understanding angles. Here are some common areas where they face challenges:
- Identifying angle types: Many students get confused when asked to classify an angle as acute, obtuse or right.
- Using a protractor: Understanding the purpose of a protractor and knowing how to use it accurately can be tricky.
- Connecting angle measures to real-world examples: Many students struggle to apply their knowledge of angles to practical situations.
To help students navigate these challenges, intervention strategies can be implemented to enhance learning:
- Hands-on activities: Engage students with activities that involve manipulating objects to form angles. For example, have students create angles using straws or string. Allow them to rotate the straws to see how the angle changes.
- Visual aids: Use posters, diagrams, or real-world examples to help students understand the different types of angles. Encourage them to draw angles and label the vertices and sides.
- **Interactive technology: Incorporate online games and simulations that involve angles. Interactive tools can make learning engaging and help students visualize concepts more effectively.
- Collaborative learning: Encourage students to work together in small groups to solve problems related to angles. Peer-to-peer learning can be incredibly beneficial in understanding and reinforcing concepts.
- **Real-Life connections: Help students explore how angles are used in their everyday lives. Ask them to identify angles in nature, architecture, or sports.
The Journey Continues: Beyond the Basics
As students progress beyond grades 1-4, they will encounter more advanced concepts related to angles, such as complementary, supplementary, and vertical angles. They will learn how to calculate missing angle measures and apply their knowledge to solve more complex geometric problems.
1-4 Study Guide And Intervention Angle Measure
Building a Foundation for Success: The Importance of an Angle-Focused Approach
Mastering angle concepts isn’t just about passing a math test; it’s a foundation for future success in various fields, from engineering and architecture to design and art. By providing students with a strong understanding of angles, we empower them to become confident problem-solvers and critical thinkers, equipped to tackle the challenges of the world around them.
It’s time to unlock the world of angles! Encourage students to explore, experiment, and apply their knowledge to real-world scenarios. And remember, even the most complex geometric problems can be solved by understanding the simple beauty of angles.






